Quantum chaos, defined as the behavior of quantum systems whose classical limits are chaotic, does not inherently lead to sensitivity to initial conditions—a hallmark of classical chaos. Surprisingly, under given circumstances, quantum-chaotic systems can display a (dynamical) phase transition, marked by a sudden shift in the system's memory behavior. This phase transition is analogous to thermodynamic phase transitions, with time acting as the temperature and the quantum expectation of the rotor's kinetic energy mimicking the free energy. Before a critical time, the system exhibits chaotic motion and loses memory of initial states; after the critical time, quantum interference enhances the likelihood of a return to the initial state, thereby recovering the system's memory.

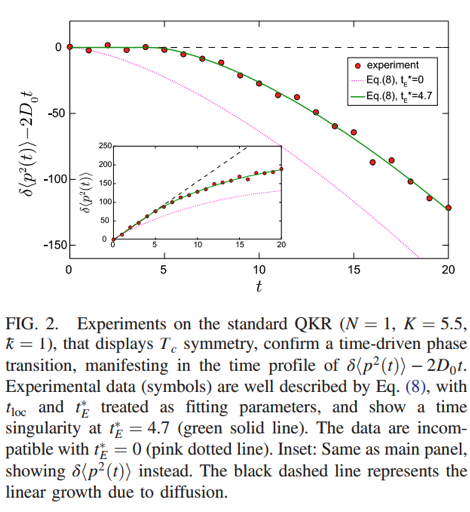
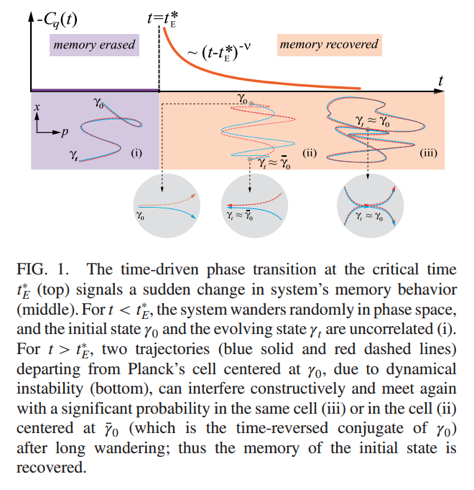
The experiment uses an atomic kicked rotor, realized with laser-cooled cloud of Cs atoms driven by short periodic pulses of a sinusoidal potential created by a far-detuned laser standing wave. By measuring the atomic momentum distribution after the application of these pulses, we observed that the rotor’s energy growth displayed a non-analyticity at a critical time, tE. This critical time, analogous to the Ehrenfest time in quantum mechanics, marks the onset of quantum interference and signifies a dramatic change in the system's memory behavior. The results demonstrate the non-trivial impact of quantum effects on chaotic systems and suggest that such time-driven phase transitions could be a universal phenomenon in quantum chaotic systems.